Michèle Cooke

Dear Friends,

I am having a rather exciting time, trying to finish working on the analysis of wave measurements on the Danube. My eager puppy approach seems to be working, albeit in a roundabout way. The other evening I told you that I imagined using a dimensionless quantity

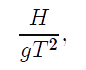
which I described as a possible "Fish distress factor", as it contains the wave height H, the wave period T, and gravitational acceleration g. I arrived at it, just because it is the only such dimensionless factor I could think of. The higher the wave, the more distress it is to fish; the shorter the wave, T small, also the larger the factor, the more distress, and all expressed relative to gravity.

Next day (probably as a result of reflecting on matters in my morning shower), things got better. I later took the simplest wave theory, Airy theory (named after the 19C Astronomer Royal in Britain, not because it is airy-fairy) and obtained the result that the highest acceleration in a short periodic wave is

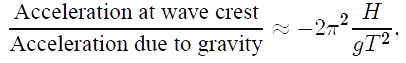
and there is the little fish distress factor, multiplied by about 20. (The highest acceleration is negative because it is at the wave crest, when your stomach wants to go through your diaphragm).

Yesterday (and this time I know it was in the shower) I suddenly realised that I do not have to go through all our records, extract waves approximately from them, and calculate the above number, based on an approximate theory (alles sehr mühsam). Instead, as we have good records of the water level height n measured at 50 times a second, I can use Fast Fourier Transforms (called by a certain New Zealand Translator and Interpreter, the “Fast and Furious Transform”) to calculate the actual acceleration of the free surface, accurately and relatively simply. And so, the Fish distress factor can be calculated as a function of time for all our wave records:

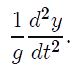
And so it is, as shown on the next page (we have 112 such pages!). I have called it the “Relative vertikale Beschleunigung” but my colleagues here couldn’t help me with a better name. As the first disturbance from the Twin City Linear arrives, shown in the second graph in red ("Wasserstand") it is still relatively slow, but the second waves and those thereafter are very short, so that the distress factor shown in the third graph is large, and at the crest of the wave has a value of about 0.8 of gravity - i.e. the acceleration in the wave is 80% of gravity. Large. Interesting.

I am very pleased. I hope my colleagues share my enthusiasm for the use of this criterion ...

Love, John

Downstream Dreamin’

Evening
Waves
Dimension-
Less
Water level
Round
About

High
Higher
Simple
Short
Morning
Shower
Reflecting
Airy
Fairy
Second
Wave
Crest

Yesterday
A function
Of slow
Time
Fast
Fourier
Furious
Transform
Extract
Imagine

Calculate,
Express.

Red disturbance
Little fish
Distress
And so
It is
Friends.

Next day
Share
Wave
Value
Gravity
Simply
Exciting
Eager
Puppy
Love.